Triangle is one of the basic geometric shapes that a child studies in their elementary math classes. It is an important math topic that finds its applications in many fields and subjects. Knowledge of this two-dimensional geometric shape and its properties is significant for children to learn other topics in trigonometry and geometry.
The area of equilateral triangle formula is applied to calculate the total space enclosed within its sides. A triangle can be classified into different types based on the length of its sides and angels. An equilateral triangle is a triangle with equal sides and angles with a measure of 60 degrees. The other two types of triangles are isosceles and scalene.
An isosceles triangle is a type of triangle in which the length of two sides and two angles are the same. A scalene triangle is one of the most common types of a triangle in which none of the sides or angles are the same. The angles of a triangle are also important to identify the type of triangle.
Based on the angles of a triangle, a triangle can be acute triangles, where all the angles are less than 90 degrees. It can be an obtuse triangle, where one of the angles is greater than 90 degrees. It can be a right triangle — one of the most prominent mathematical shapes inspiring Pythagoras’ Theorem and trigonometry.
The Pythagoras or Pythgoras’ theorem is used to find the length of a side when the length of other two sides are provided. The area of right triangle is calculated using the formula ½ x Base x Height.
Properties of Equilateral Triangles
- An equilateral triangle is a regular polygon with three sides and three vertices.
- All three sides of an equilateral triangle are equal.
- All the angles of an equilateral triangle are congruent and equal to 60 degrees.
- The perpendicular from one corner of an equilateral triangle to the opposite side bisects it into equal halves. Also, the angle at the vertex from which the perpendicular is drawn is divided into two equal angles, i.e., 30 degrees each.
- The ortho-center and centroid of an equilateral triangle are at the same point.
- The median, angle bisector, and altitude for all sides are all the same in an equilateral triangle.
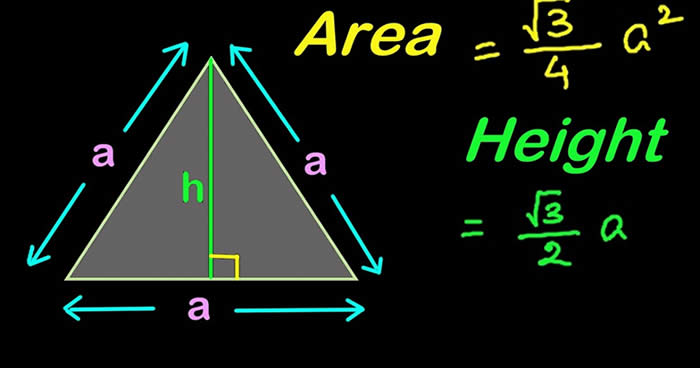
- The formula used to calculate the area of an equilateral triangle is √3/4 ×(side)2.
- The formula used to calculate the perimeter of an equilateral triangle is 3a, where a is the length of a side.
Area of an Equilateral Triangle
Calculating the area of a triangle is an important skill used by many professionals in different fields. The total space enclosed within its sides of a two-dimensional equilateral triangle is called the area of an equilateral triangle.
It is calculated by using the formula √3/4 × (side)2. For example, if the length of a side of an equilateral triangle is 2 cm then the area of this triangle can be √3/4 × (2)2 = √3 sq. centimeter.
Application & Importance of Equilateral Triangles
An equilateral triangle is not just mathematically important; it is also significant to the way we build structures and foundations, both physically and virtually. The equilateral triangle is one of the most common types of triangles used in architecture.
An equilateral triangle has three congruent sides and angles measuring 60 degrees on each corner. Equilateral triangles are special because they are strong. A well-known application of equilateral triangles in architecture is the Pyramids in Egypt. Each side of the pyramids is an equilateral triangle– the testimony of the strength of the triangles in architecture.
Among the various two-dimensional shapes that are built from metal struts, the triangle is the strongest. All other shapes can be distorted with a simple force if they are hinged at the corners.
But not the trusty triangle, which explains its extensive use in the field of architecture and construction, from pylons to bracing. By applying the concepts of perimeter and area of a triangle we can determine the various dimensions of such a building or a structure.